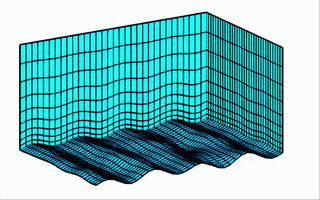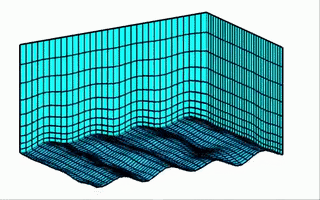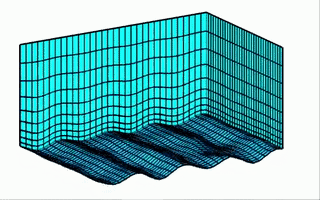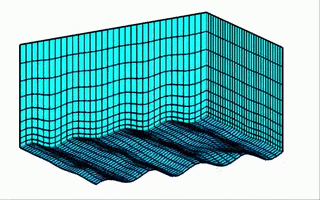
To study the viscous and turbulent flows with undulating boundaries, such as the water waves and wavy walls, we have developed a numerical method that simulates the Navier–Stokes equations on a time-dependent boundary-fitted grid, i.e. using the Arbitrary Lagrangian-Eulerian (ALE) method on moving grid. The governing equations are transformed into computational curvilinear coordinates and are written using a strong conservative formulation, which ensures a good conservation of mass and momentum. The equations are discretized by a hybrid pseudo-spectral and finite-difference method, which is highly accurate and efficient. For free-surface flows, fully nonlinear kinematic and dynamic boundary conditions are implemented to capture the flow physics at the free surface. For high Reynolds number turbulent flows, large-eddy simulation (LES) is implemented using a scale-dependent Lagrangian-averaged dynamic model for subgrid-scale stress. This model addresses the heterogeneity near the boundary with complex geometries, such as waves. Our group has used this numerical method in a variety of studies, including turbulent wind over waves, free-surface turbulence, and wave-current-turbulence interaction.
Selected Publications:
- Xuan, A. & Shen, L. (2019), “A conservative scheme for simulation of free-surface turbulent and wave flows,” Journal of Computational Physics, Vol. 378, pp.18-43.
- Yang, D. & Shen, L. (2011), “Simulation of viscous flows with undulatory boundaries: Part II. Coupling with other solvers for multi-fluids computation,” Journal of Computational Physics, Vol. 230, pp.5510-5531.
- Yang, D. & Shen, L. (2011), “Simulation of viscous flows with undulatory boundaries: Part I. Basic solver,” Journal of Computational Physics, Vol. 230, pp.5488-5509.